No products in the cart.

Translation in BIOZONE WORLD
Read more about how we are supporting ELL students in the classroom and introducing translation into BIOZONE WORLD.
BIOZONE is excited to announce the launch of BIOZONE WORLD. A stunning new science content delivery platform that brings together all of our digital resources for easy access.
Our resources are unlike any you’ve seen before, and a departure from the basal textbook paradigm.
We take a WorkText approach, combining the very best features of a traditional textbook with the utility of a workbook.

Read more about how we are supporting ELL students in the classroom and introducing translation into BIOZONE WORLD.

As we approach the festive season, we want to express our sincere gratitude for your continued support throughout the year. It has been a pleasure working with you, and we’re excited about the possibilities the coming year holds.

If you missed attending any conferences and joining our workshops, don’t worry we have all you need to know here.

Read more about how we are supporting ELL students in the classroom and introducing translation into BIOZONE WORLD.

Read more about how we are supporting ELL students in the classroom and introducing translation into BIOZONE WORLD.

As we approach the festive season, we want to express our sincere gratitude for your continued support throughout the year. It has been a pleasure working with you, and we’re excited about the possibilities the coming year holds.
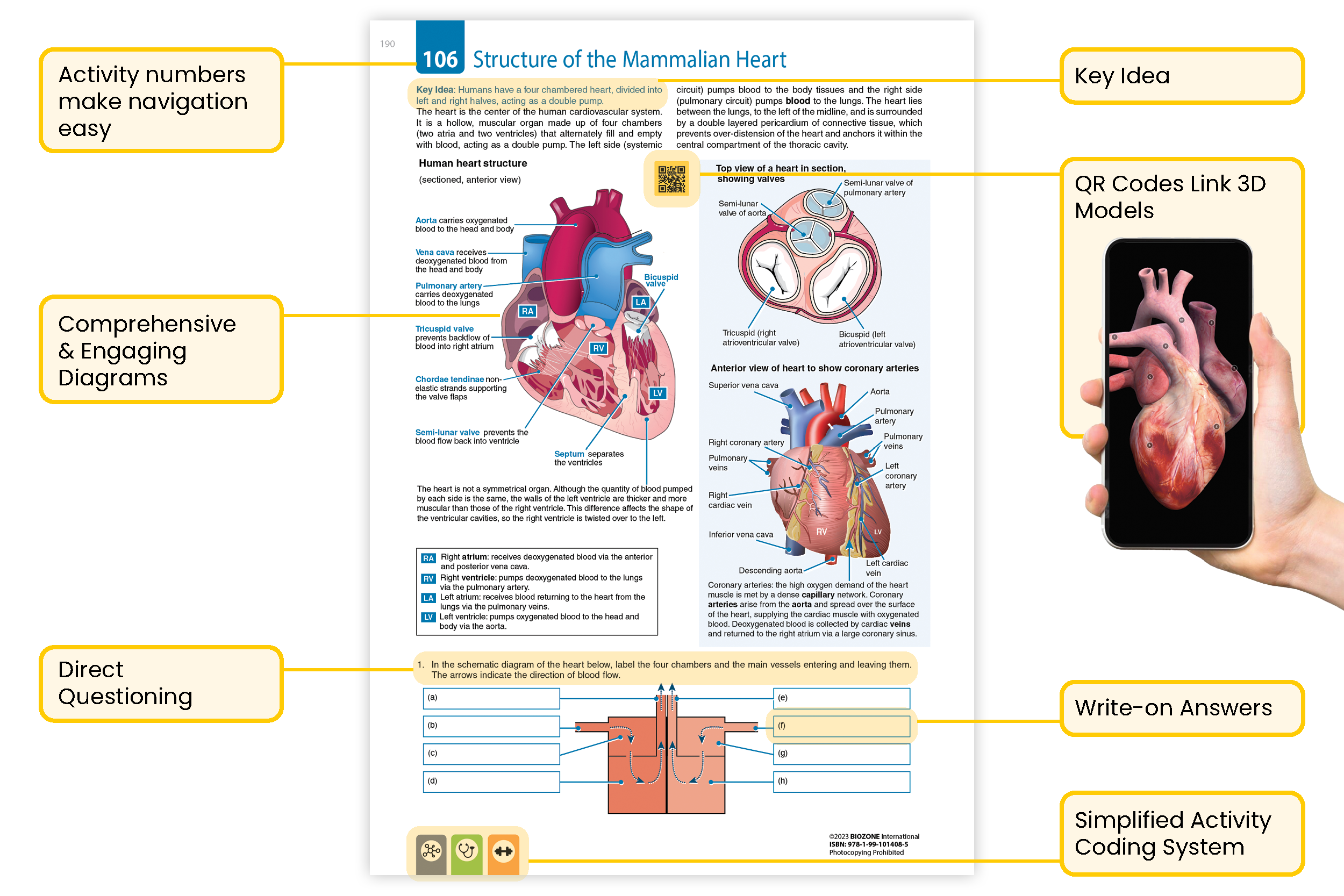
BIOZONE’s unique, interactive worktext approach encourages direct interaction with the content, allowing students to record their answers within the context of the stimulus material and form a record of work for quick and easy revision.
Get in touch with our team now to arrange a call

By their innovative design, our resources encourage student interaction, using simple investigations and data analysis to engage students in the science around them
** Conditions apply. Available on application. Integration to most popular Learning Management Systems.
Flexible and cost-effective in-person, virtual/webinar sessions are available with our trainer across the United States or globally.
Let us tailor solutions for your teachers!

Explore what sets us apart from our competitors and why our resources and solutions are perfect for your school.
Each program is available in both print and digital formats.



Get in contact with us to have a catalog sent to you or view online now by clicking below
